Sorry this page is restricted to Basic members and above. Please log in below or join.
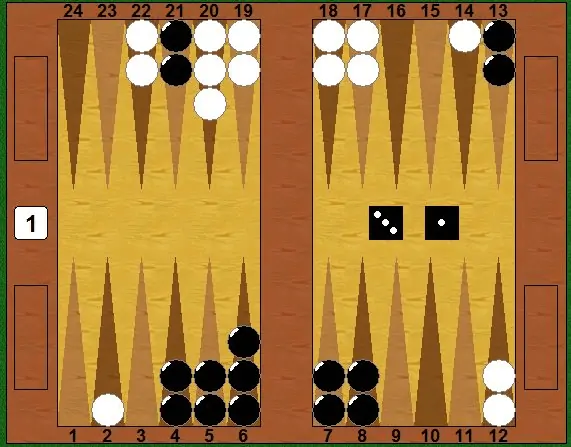
Bill Robertie challenge #10
Bill Robertie Challenge — A Great Member Benefit
The Bill Robertie Challenge is a series of 158 problems illustrating key backgammon principles.
Take the challenge and improve your game!
- Tagged: Doubling Decision